Statistics Tip of the Week When comparing p to Alpha, or a Test Statistic value to the Critical value, which way does the "<" point?

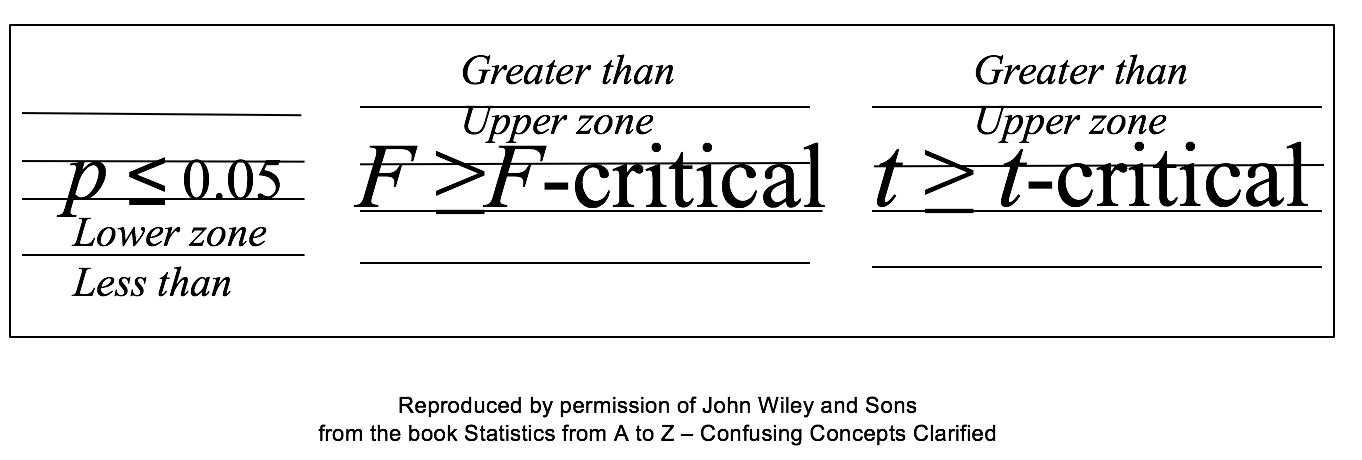
We run into p and Test Statistics – such as t, F, z, and χ2 – in a number of statistical tests, such as t-tests, F-tests, and ANOVA. After performing one of these tests, we come to a conclusion based on whether p ≤ or > 0.05 (or other value for Alpha) – or whether t < or > t-critical.

But beginners can sometimes forget which way the "<" or ">" is supposed to point:
​
Does p < Alpha tells us that there is or is not a Statistically Significant difference?
 
The following is a non-statistical gimmick, but it may be helpful for some – it was for me. In this book, we don't focus on confusing things like the "nothingness" of the Null Hypothesis. We focus on something that does exist – like a difference, a change or an effect. So, to make things easy, we want a memory cue that tells us when there is something, as opposed to nothing.
 
We can come to the following conclusions (depending on the test):

Q:  But how do we remember which way the inequality symbol should go? The book gives 3 rules and a statistical explanation and the following visual cue: 
​
Remember back in kindergarten or first grade, when you were learning how to print?  The letters of the Alphabet were aligned in 3 zones – middle, upper, and lower as below.
 
p is different from t or F, because p extends into the lower zone, while F, t, and χ2 extend into the upper zone. (z doesn't; it stays in the middle zone. But we can remember that z is similar to t.)
 
If we associate the lower zone with less than, and the upper zone with greater than, we have the following memory cue: