What's the Difference between a 2-Sample t-test and a Paired t-test?

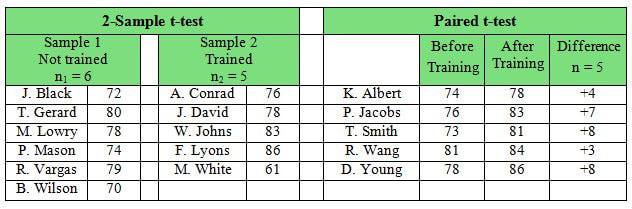
​Many folks are confused about this, especially since the names for these tests themselves can be misleading. What we're calling the "2-Sample t-test" is sometimes called the "Independent Samples t-test". And what we're calling the "Paired t-test" is then called the "Dependent Samples t-test", implying that it involves more than one Sample. But that is not the case. It is more accurate -- and less confusing -- to call it the  Paired t-test.

First of all, notice that the 2-Sample test, on the left, does have 2 Samples. We see that there are two different groups of test subjects involved (note the names are different) -- the Trained and the Not Trained. The 2-Sample t-test will compare the Mean score of the people who were not trained with the Mean score of different people who were trained.

The story with the Paired Samples t-test is very different. We only have one set of test subjects, but 2 different conditions under which their scores were collected. For each person (test subject), a pair of scores -- Before and After -- was collected. (Before-and-After comparisons appear to be the most common use for the Paired test.) 

Then, for each individual, the difference between the two scores is calculated. The values of the differences are the Sample (in this case: 4, 7, 8, 3, 8 ). And the Mean of those differences is compared by the test to a Mean of zero.

For more on the subject, you can view my video, t, the Test Statistic and its Distributions.      
​